Cocktail Sort

C Program executes cocktail sort.

Combo sort is a somewhat straightforward arranging calculation initially planned by Wlodzimierz Dobosiewicz and Artur Borowy in 1980, later rediscovered by Stephen Lacey and Richard Box in 1991.

Combo sort enhances bubble sort similarly that Shellsort enhances inclusion sort.

cocktail sort is a variety of air pocket sort that is both a steady arranging calculation and an examination sort.

The calculation contrasts from an air pocket sort in that it figures out in the two headings on each pass the rundown.

This arranging calculation is simply hardly more challenging to execute than an air pocket sort, and takes care of the issue of turtles in bubble sorts.

The different names of mixed drink sort are bidirectional cocktail sort, mixed drink shaker sort, cocktail sort , swell sort, mix sort, transport sort or party time sort

Here is source code of the C Program to execute cocktail sort. The C program is effectively incorporated and run on a Linux framework.

The program yield is likewise displayed underneath.

The mixed drink shaker sort is an enhancement for the Bubble Sort.

The improvement is fundamentally that qualities "bubble" the two bearings through the exhibit, in light of the fact that on every emphasis the cocktail sort bubble sorts once advances and once in reverse.

 Pseudocode for the calculation

capability cocktailSorting( A : rundown of sortable things )
 do
   swap:= true
   for every I in 0 to length( A ) - 2 do
     on the off chance that A[ I ] > A[ i+1 ],//test whether the two
                               // components are off base
                               // request
       swap( A[ I ], A[ i+1 ] )//let the two components
                                // change places
       swap := true;
   in the event that swap = false,
     // we can leave the external circle here assuming no trades happened.
     break do-while loop;
   swap := false
   for every I long( A ) - 2 down to 0 do
     in the event that A[ I ] > A[ i+1 ],
       swap( A[ I ], A[ i+1 ] )
       swap := true;
 while swaped;//in the event that no components have been traded,
                // then the rundown is arranged

Cocktail sort is the variety of Bubble Sort, which crosses the rundown in the two bearings on the other hand.

 It is not the same as air pocket sort as in bubble sort navigates the rundown in the forward heading just, while this calculation crosses in forward as well as in reverse course in one emphasis.

Cocktail sort is likewise called as bi-directional bubble sort. In bubble sort, components are navigated from left to right, i.e., in one course.

In first cycle, bubble sort first moves the biggest component to its right position, then, at that point, the second-biggest component in its accurate position, etc. Be that as it may, mixed drink sort crosses in the two headings on the other hand.

As like the bubble sort, the most terrible and normal case of cocktail sort is O(n2). Cocktail sort is quicker than bubble sort.

There are 2 stages in this sorting

1. In first stage, like the bubble sort, loop through the cluster from left to right. The nearby components are analyzed, and assuming the left component is more prominent than the right one, we swap those components. The biggest component of the rundown is set toward the finish of the exhibit in the forward pass.

2.In second stage, loop through the cluster from the furthest right unsorted component to the left. The neighboring components are analyzed, and on the off chance that the right component is more modest than the left component, we trade those components. The littlest component of the rundown is put toward the start of the cluster in the regressive pass.

This loop goes on until the elements are sorted.

Complexities:

Best Case Complexity - It happens when there is no arranging required, i.e., the array is now arranged. The best-case time complexity of cocktail sort is O(n).

Average Case Complexity - It happens when the array components are in confused request that isn't as expected climbing and not appropriately plummeting. The average case time complexity of cocktail sort is O(n2).

Worst Case Complexity - It happens when the array elemets are expected to be arranged backward request. That implies guess you need to sort the array elements in rising request however, its components are in diving request. The worstcase time complexity of cocktail sort is O(n2).

Space Complexity – the space complexity of cocktail sorting is O(1)

 Example for cocktail sorting:

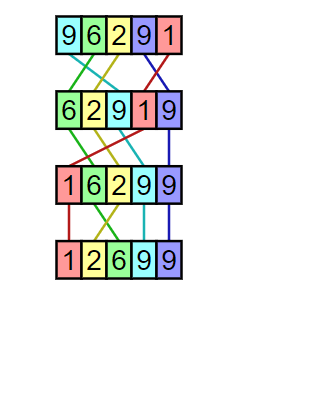
#When its fast

Mixed drink sort is at its quickest when it can arrive at an arranged rundown with an insignificant number of passes.

Since just adjoining components are traded, this implies that mixed drink sort performs best when components are actually close by their arranged positions.

Comparision with bubble sort:

1.Time complexities are same, yet Cocktail performs better compared to Bubble Sort. Normally cocktail sort is under twice quicker than bubble sort.

2.As the cocktail sort goes bidirectionally, the scope of potential trades, which is the reach to be tried, will diminish per pass, in this way decreasing the general running time marginally.

// Java program for execution of Cocktail Sort
public class CocktailSort1
{
	void cocktailSort(int a[])
	{
		boolean swapped = true;
		int start = 0;
		int end = a.length;


		while (swap == true)
		{
			// reset the traded banner on entering the
			// circle, since it very well may be valid from a
			// past cycle.
			Swapped = false;


			// circle from base to top same as
			// the cocktail sort
			for (int i = begin; i< end - 1; i++)
			{
				int (a[i] > a[i + 1]) {
					int temp = a[i];
					a[i] = a[i + 1];
					a[i + 1] = temp;
					swapped = true;
				}
			}


			// in the case of nothing moved, then, at that point, exhibit is arranged.
			if (traded == bogus)
				break;


			// in any case, reset the traded banner so it
			// can be utilized in the following stage
			swapped = false;


			// move the end point back by one, in light of the fact that
			// thing toward the end is in its legitimate spot
			end = end - 1;


			// start to finish, doing the
			// same examination as in the past stage
			for (int I = end - 1; I >= begin; I- - )
			{
				on the off chance that (a[i] > a[i + 1])
				{
					int temp = a[i];
					a[i] = a[i + 1];
					a[i + 1] = temp;
					traded = valid;
				}
			}


			// increment the beginning stage, on the grounds that
			// the last stage would have moved the following
			// most modest number to its legitimate spot.
			begin = begin + 1;
		}
	}


	/* Prints the exhibit */
	void printArray(int a[])
	{
		int n = a.length;
		for (int I = 0; I < n; i++)
			System.out.print(a[i] + " ");
		System.out.println();
	}

// Driver code

public static void main(String[] args)
	{
		CocktailSort ob = new CocktailSort();
		int a[] = { 5, 1, 4, 2, 8, 0, 2 };
		ob.cocktailSort(a);
		System.out.println("Sorted exhibit");
		ob.printArray(a);
	}
}