Data Structures Tutorial

Data Structures Tutorial Asymptotic Notation Structure and Union Array Data Structure Linked list Data Structure Type of Linked list Advantages and Disadvantages of linked list Queue Data Structure Implementation of Queue Stack Data Structure Implementation of Stack Sorting Insertion sort Quick sort Selection sort Heap sort Merge sort Bucket sort Count sort Radix sort Shell sort Tree Traversal of the binary tree Binary search tree Graph Spanning tree Linear Search Binary Search Hashing Collision Resolution Techniques

Misc Topic:

Priority Queue in Data Structure Deque in Data Structure Difference Between Linear And Non Linear Data Structures Queue Operations In Data Structure About Data Structures Data Structures Algorithms Types of Data Structures Big O Notations Introduction to Arrays Introduction to 1D-Arrays Operations on 1D-Arrays Introduction to 2D-Arrays Operations on 2D-Arrays Strings in Data Structures String Operations Application of 2D array Bubble Sort Insertion Sort Sorting Algorithms What is DFS Algorithm What Is Graph Data Structure What is the difference between Tree and Graph What is the difference between DFS and BFS Bucket Sort Dijkstra’s vs Bellman-Ford Algorithm Linear Queue Data Structure in C Stack Using Array Stack Using Linked List Recursion in Fibonacci Stack vs Array What is Skewed Binary Tree Primitive Data Structure in C Dynamic memory allocation of structure in C Application of Stack in Data Structures Binary Tree in Data Structures Heap Data Structure Recursion - Factorial and Fibonacci What is B tree what is B+ tree Huffman tree in Data Structures Insertion Sort vs Bubble Sort Adding one to the number represented an array of digits Bitwise Operators and their Important Tricks Blowfish algorithm Bubble Sort vs Selection Sort Hashing and its Applications Heap Sort vs Merge Sort Insertion Sort vs Selection Sort Merge Conflicts and ways to handle them Difference between Stack and Queue AVL tree in data structure c++ Bubble sort algorithm using Javascript Buffer overflow attack with examples Find out the area between two concentric circles Lowest common ancestor in a binary search tree Number of visible boxes putting one inside another Program to calculate the area of the circumcircle of an equilateral triangle Red-black Tree in Data Structures Strictly binary tree in Data Structures 2-3 Trees and Basic Operations on them Asynchronous advantage actor-critic (A3C) Algorithm Bubble Sort vs Heap Sort Digital Search Tree in Data Structures Minimum Spanning Tree Permutation Sort or Bogo Sort Quick Sort vs Merge Sort Boruvkas algorithm Bubble Sort vs Quick Sort Common Operations on various Data Structures Detect and Remove Loop in a Linked List How to Start Learning DSA Print kth least significant bit number Why is Binary Heap Preferred over BST for Priority Queue Bin Packing Problem Binary Tree Inorder Traversal Burning binary tree Equal Sum What is a Threaded Binary Tree? What is a full Binary Tree? Bubble Sort vs Merge Sort B+ Tree Program in Q language Deletion Operation from A B Tree Deletion Operation of the binary search tree in C++ language Does Overloading Work with Inheritance Balanced Binary Tree Binary tree deletion Binary tree insertion Cocktail Sort Comb Sort FIFO approach Operations of B Tree in C++ Language Recaman’s Sequence Tim Sort Understanding Data Processing Applications of trees in data structures Binary Tree Implementation Using Arrays Convert a Binary Tree into a Binary Search Tree Create a binary search tree Horizontal and Vertical Scaling Invert binary tree LCA of binary tree Linked List Representation of Binary Tree Optimal binary search tree in DSA Serialize and Deserialize a Binary Tree Tree terminology in Data structures Vertical Order Traversal of Binary Tree What is a Height-Balanced Tree in Data Structure Convert binary tree to a doubly linked list Fundamental of Algorithms Introduction and Implementation of Bloom Filter Optimal binary search tree using dynamic programming Right side view of binary tree Symmetric binary tree Trim a binary search tree What is a Sparse Matrix in Data Structure What is a Tree in Terms of a Graph What is the Use of Segment Trees in Data Structure What Should We Learn First Trees or Graphs in Data Structures All About Minimum Cost Spanning Trees in Data Structure Convert Binary Tree into a Threaded Binary Tree Difference between Structured and Object-Oriented Analysis FLEX (Fast Lexical Analyzer Generator) Object-Oriented Analysis and Design Sum of Nodes in a Binary Tree What are the types of Trees in Data Structure What is a 2-3 Tree in Data Structure What is a Spanning Tree in Data Structure What is an AVL Tree in Data Structure Given a Binary Tree, Check if it's balanced B Tree in Data Structure Convert Sorted List to Binary Search Tree Flattening a Linked List Given a Perfect Binary Tree, Reverse Alternate Levels Left View of Binary Tree What are Forest Trees in Data Structure Compare Balanced Binary Tree and Complete Binary Tree Diameter of a Binary Tree Given a Binary Tree Check the Zig Zag Traversal Given a Binary Tree Print the Shortest Path Given a Binary Tree Return All Root To Leaf Paths Given a Binary Tree Swap Nodes at K Height Given a Binary Tree Find Its Minimum Depth Given a Binary Tree Print the Pre Order Traversal in Recursive Given a Generate all Structurally Unique Binary Search Trees Perfect Binary Tree Threaded Binary Trees Function to Create a Copy of Binary Search Tree Function to Delete a Leaf Node from a Binary Tree Function to Insert a Node in a Binary Search Tree Given Two Binary Trees, Check if it is Symmetric A Full Binary Tree with n Nodes Applications of Different Linked Lists in Data Structure B+ Tree in Data Structure Construction of B tree in Data Structure Difference between B-tree and Binary Tree Finding Rank in a Binary Search Tree Finding the Maximum Element in a Binary Tree Finding the Minimum and Maximum Value of a Binary Tree Finding the Sum of All Paths in a Binary Tree Time Complexity of Selection Sort in Data Structure How to get Better in Data Structures and Algorithms Binary Tree Leaf Nodes Classification of Data Structure Difference between Static and Dynamic Data Structure Find the Union and Intersection of the Binary Search Tree Find the Vertical Next in a Binary Tree Finding a Deadlock in a Binary Search Tree Finding all Node of k Distance in a Binary Tree Finding Diagonal Sum in a Binary Tree Finding Diagonal Traversal of The Binary Tree Finding In-Order Successor Binary Tree Finding the gcd of Each Sibling of the Binary Tree Greedy Algorithm in Data Structure How to Calculate Space Complexity in Data Structure How to find missing numbers in an Array Kth Ancestor Node of Binary Tree Minimum Depth Binary Tree Mirror Binary Tree in Data Structure Red-Black Tree Insertion Binary Tree to Mirror Image in Data Structure Calculating the Height of a Binary Search Tree in Data Structure Characteristics of Binary Tree in Data Structure Create a Complete Binary Tree from its Linked List Field in Tree Data Structure Find a Specified Element in a binary Search Tree Find Descendant in Tree Data Structure Find Siblings in a Binary Tree Given as an Array Find the Height of a Node in a Binary Tree Find the Second-Largest Element in a Binary Tree Find the Successor Predecessor of a Binary Search Tree Forest of a Tree in Data Structure In Order Traversal of Threaded Binary Tree Introduction to Huffman Coding Limitations of a Binary Search Tree Link State Routing Algorithm in Data Structure Map Reduce Algorithm for Binary Search Tree in Data Structure Non-Binary Tree in Data Structure Quadratic Probing Example in Hashing Scope and Lifetime of Variables in Data Structure Separate Chaining in Data Structure What is Dynamic Data Structure Separate Chaining vs Open Addressing Time and Space Complexity of Linear Data Structures Abstract Data Types in Data Structures Binary Tree to Single Linked List Count the Number of Nodes in the Binary Tree Count Total No. of Ancestors in a Binary Search Tree Elements of Dynamic Programming in Data Structures Find cost of tree with prims algorithm in data structures Find Preorder Successor in a Threaded Binary Tree Find Prime Nodes Sum Count in Non-Binary Tree Find the Right Sibling of a Binary Tree with Parent Pointers Find the Width of the Binary Search Tree Forest trees in Data Structures Free Tree in Data Structures Frequently asked questions in Tree Data Structures Infix, Postfix and Prefix Conversion Time Complexity of Fibonacci Series What is Weighted Graph in Data Structure What is the Advantage of Linear Search?

Minimum Depth Binary Tree
2023-03-19 23:39:21

Minimum Depth Binary Tree

The depth of the binary tree is the number of levels of tree where the nodes elements are present on tree data structure. The minimum depth of a binary tree is the shortest distance from the root node to a leaf node. The minimum depth is also known as the minimum height of the tree. The minimum depth of a binary tree is an important property of the tree because it can affect the performance of certain tree traversal algorithms, such as breadth-first search. A tree with a small minimum depth will have a larger minimum depth than a tree with a large minimum depth, which means that it will take longer to reach the leaf nodes in the tree. This can have an impact on the time complexity of the algorithm. The minimum depth of a binary tree is also important because it can be used to determine the balance of the tree. A tree with a small minimum depth may be unbalanced, with most of the nodes concentrated at the top of the tree. On the other hand, a tree with a large minimum depth may be more balanced, with nodes distributed more evenly throughout the tree.

The minimum depth of a binary tree is defined as the shortest distance from the root node to a leaf node, where a leaf node is defined as a node that has no children. In other words, it is the length of the path from the root node to the nearest leaf node. This can be computed using a breadth-first search or a depth-first search algorithm, which visits the nodes of the tree level by level or node by node, respectively. It has various applications in computer science, such as determining the balance of a tree and providing a way to optimize tree-based algorithms by pruning branches that do not lead to any useful information. In general, a balanced binary tree is one where the minimum depth and the maximum depth differ by at most 1, which ensures that the tree is not skewed to one side and can be searched efficiently.

The minimum depth of a binary tree is equal to the number of edges in the shortest path from the root to a leaf node. The minimum depth of a binary tree is also equal to the number of nodes in the shortest path from the root to a leaf node, minus one. This is because the minimum depth counts the number of edges, and each edge connects two nodes, so the minimum depth is equal to the (number of nodes- 1)

For example, in the following binary tree the minimum depth is 2, because the shortest path to a leaf node is through the nodes with values 3 and 4:

   1
  / \
 2   3
/    \
4     5

In a binary tree with only one node (the root), the minimum depth is 1. In an empty binary tree (a tree with no nodes), the minimum depth is 0.

It is possible to calculate the minimum depth of a binary tree using a depth-first search or a breadth-first search. In a depth-first search, we traverse the tree by exploring as far as possible down each branch before backtracking. In a breadth-first search, we traverse the tree by visiting all the nodes at a given depth before moving on to the nodes at the next depth.

Minimum Depth Binary Tree

Figure: Minimum depth of Binary tree

  1
 /  \
 2   3
/    \
4      5

Another example of the minimum depth of the binary tree is as in above structure as the minimum depth of this binary tree is 2. The shortest path from the root node (1) to a leaf node (4 or 5) is 2, which includes the nodes 1, 2, and either 4 or 5.

How to find Minimum Depth in Binary Tree

To find the minimum depth, we can traverse the tree using a breadth-first search or a depth-first search algorithm and keep track of the current depth. We start at the root node with a depth of 1 and then visit the children of the current node. Whenever we reach a leaf node, we compare its depth with the current minimum depth and update it if it is smaller.

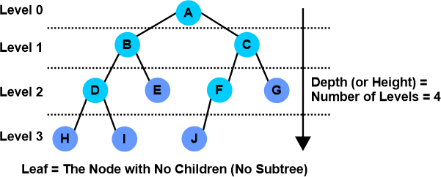
Minimum Depth Binary Tree

Figure: View of minimum depth of Binary Tree

Overall, the ideal minimum depth for a binary tree will depend on the specific use case and the requirements of the application. It may be necessary to balance the trade-off between the time complexity of the algorithm and the space complexity of the tree in order to find the optimal solution. A tree with a large minimum depth may be more balanced, with nodes distributed more evenly throughout the tree. This can make it faster to reach the leaf nodes, which can improve the performance of the algorithm.

There are a few properties of the minimum depth of a binary tree that are, the minimum depth of a binary tree with n nodes is at most log (n), where log is the base 2 logarithm. This is because in a perfectly balanced binary tree, the minimum depth will be log(n), since the tree will have log(n) levels and each level will have 2^level node.