What are the types of Trees in Data Structure

Data structures

Data management is called database management. This allows the computer to sort or organize the data for efficient retrieval. A data model is a system used to store, manage, and optimize computer resources. Data processing is not just about data storage. Almost every app or program has notices and updates about its version. Data structures are so simple and complex that it is difficult to program with a programming language that does not have data structures.

Data processing is the process of using intelligence and software to manage, organize, store and store information on a computer device or system. Data models provide visualization for easy data organization and management. Any basic process, program, or program has two parts: data and algorithms - the rules and regulations of data exchange and algorithms.

Linear Data structures

This data type adds data to the data type. It's all about the process. You can then delete the duplicate. There are four types of linear data, they are:

1. Queue
2. Stack
3. Linked lists
4. Array

Non-linear Data structures

Data formats can be created in a variety of ways.

1. Tree data structure
2. Graph data structure

Tree Data structure

The information about the tree is self-explanatory. Trees are ordered and, therefore, not linear. But they are actually designed differently.

Tree A node-based data model that represents and supports the structure as a structure. In an asynchronous database, data is stored in a tree data structure called a database. Each line of text is called the lower branch of the data type tree.

Two types of plants. This is limited to confidential information. Because this data processing is an individual process. This means that a binary plant can produce 0, 1, or 2 seeds at any time. Binary search trees can quickly parse nested and linked expressions. This allows binary trees to provide values ??from list and associate arrays. This makes it easier to find hidden items. (because it is a powerful data structure)

Tree terminology in data structures

Common Object Data Structure Concept Map. These general principles are often used in conceptual data classification.

1. Child
2. Parent
3. Edge
4. Root
5. Node
6. External nodes
7. Internal nodes
8. Leaf
9. Neighbor node
10. Siblings
11. Height
12. Level
13. Degree
14. Descendants
15. Ancestors
16. Subtree
17. Path
18. Depth

Properties of a tree

1. Number of edges – A connection between two nodes in a tree data structure is called an edge. There is only one path between two file tree nodes. A positive number in this data structure is equal to -1 if all nodes in the tree data structure are equal.
2. The circle level is the sum of all subtrees defined in the tree data structure of the selected node, called the node level. The root node level (also known as the tree level) should be the highest level relative to other nodes in the tree data structure. The leaf level of the data tree must be zero (0).
3. Tree Level – The root node level is the tree level.

 Applications of trees

Trees are often used for data processing. Some of them are mentioned below.

1. Keys can be arranged in a tree (e.g., BST (Binary Search Tree)). This tree makes listing links easy and quick. However, the comparison will be simple. Black and red forage plants and AVL plants (Adelson, Velsky, Landis) were evaluated independently. This ensures that O(log N) searches are stored.
2. Red, black, and AVL trees (Adelson, Velsky, and Landis trees) are independent search trees at O(log n) coverage or low coverage. You can delete or assign keys if you want. However, the comparison will be simple.
3. Use data charts and graphs similar to linked lists. But unlike structures, it means that vertices are connected by arrows, and there are no borders.
4. Data is stored in a document tree and document format (HTML, XML, etc.). The generated data is stored in this file.
5. Ideal for rapid access, retrieval, and retrieval of production data. Get a Binary Search Tree (BST).
6. Wood is used to build houses and other things. For general and building equipment
7. Data centers use b-trees and b-trees for classification.
8. Grammar maps are used for structural, visual, and quantitative analysis.
9. A K-D tree as tree is a type of prediction tree. A data map organizes these objects into a space of width K.
10. A tree is a piece that uses a dictionary to look up topics.
11. Organizational concepts can be easily obtained with the help of statistical tables.
12. Look at wood as a new and attractive model.
13. A prediction tree is used as a decision tree.
14. Large companies use data charts as organizational charts.
15. XML files are used for parsing.
16. Data trees are used to write algorithms for machine learning models.
17. Multiple servers are used, such as domain name servers (DNS).
18. File trees can be used to create computer models.
19. Course Evaluation
20. Data trees prevent players from playing football.
21. Java Virtual Machine (JVM).

Types of trees in data structures

Now that we know what trees are let us learn the different types of trees in data structures.

There are six types of trees in data structures, and they are mentioned below:

1. General tree
2. Binary tree
3. Binary search tree
4. AVL tree (Adelson, Velskii, & Landis Tree)
5. Red-Black tree
6. N array tree

General trees

A tree is said to be a general tree when there are no constraints (rules) on the hierarchy of the Tree. The general trees are considered the superset of all the types of tree present in data structures. The nodes in the general trees can have child nodes counting from 0 to infinity. This means there is no restriction on the number of child nodes any parent node in the Tree can have.

Here is an example of a general tree:

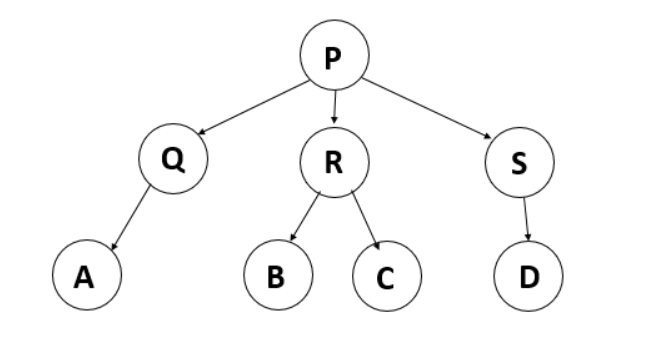
We can observe that the above-shown general Tree has no rules in its hierarchy.

Binary trees

The binary trees are the most popular trees among all the other trees in data structures. These types of trees have constraints (rules) on their hierarchy. All the nodes of a binary tree either have two or zero (0) child nodes.

Here is an example of a binary tree:

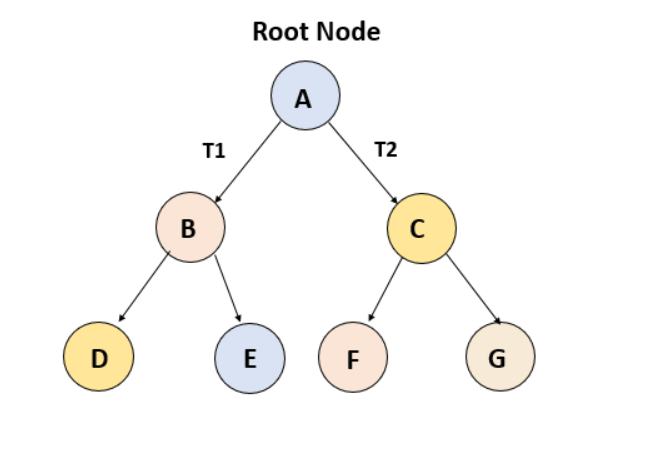
We can observe that each node in the above Tree has a maximum of only two child nodes.

Binary Search tree

BST, also known as a binary search tree, is considered an extension of binary trees. It includes several other restrictions and constraints that make a binary tree. In a binary search tree (BST), the left child node of every node must be less than or equal to the node itself (parent node), and the right child node of every node must be greater than or equal to the node itself (parent node). As we design the Tree such that the left subtree of any node in the Tree consists of the values less than or equal to the node itself (parent node), and the right subtree of the selected node consists of the values greater than or equal to the node itself (parent node), Binary search trees (BST) are ideal for performing search operations. This is the reason behind naming the Tree as such.

Here is an example of a binary search tree:

We can observe that in the above Tree, we have all the left child nodes less than their respective parent nodes and their right child nodes are greater than their respective parent nodes.

AVL tree (Adelson, Velskii, & Landis Tree)

The AVL tree (Adelson, Velskii, and Landis tree) is the first dynamically balanced Tree. It is a type of binary search self-balancing tree.

Here is an example of an AVL tree (Adelson, Velskii, and Landis tree):

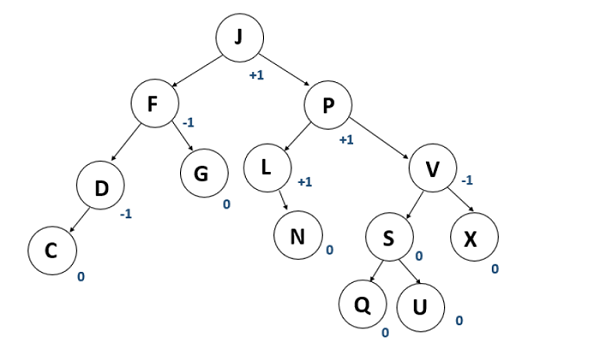
We can observe that the above Tree has all the features of an AVL tree (Adelson, Velskii, and Landis tree).

Red-black tree

Red-black Tree can be considered another example of a self-balancing tree. As the name suggests, all the nodes of a red-black tree are either painted red or black. The red-black Tree maintains the balance of the forest. The Red Black Tree is not fully balanced. It takes an O (log N) period to perform a searching operation in a Red-black tree.

Here is an example of a Red-black Tree:

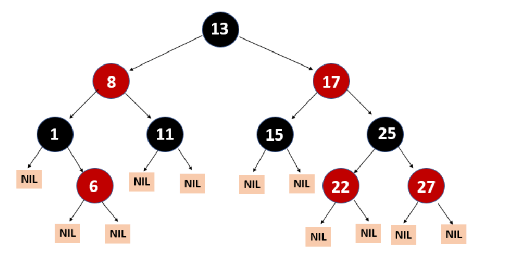
We can observe that the above Tree has all the features of a Red-black Tree.

N–ary tree

The N-ary Tree has a maximum of N number of child nodes. For example, a binary tree is considered a 2 – year tree as each node can have a maximum of two nodes.

Here is an example of N – ary tree (binary Tree):

The N-ary Tree has a maximum of N number of child nodes. For example, a binary tree is considered a 2 – year tree as each node can have a maximum of two nodes.

Here is an example of N – ary tree (binary Tree):

Here is an example of N – ary tree (binary Tree):

We can observe that in the above binary Tree, each node has a maximum of two child nodes (two-year tree).