Quick Sort

Quicksort is a sorting algorithm that uses a divide-and-conquer strategy.

1. A pivot element is used to divide an array into subarrays (element selected from the array).  The pivot element should be positioned so that elements less than pivot are kept on the left side of the pivot and elements greater than pivot are kept on the right side of the pivot when dividing the array.
2. The same method is used to divide the left and right subarrays. This process is repeated until each subarray has only one element.
3. The elements have already been sorted at this point. The elements are finally able to produce a sorted array.

The Quicksort Algorithm Steps

1. Choose the Pivot Element.

Quicksort can be done in a variety of ways, with the pivot element being chosen from a variety of positions. The rightmost element of the array will be used as the pivot element in this case.

2. Arrange the Array in a new order.

The array's elements are now rearranged so that elements smaller than the pivot are on the left and elements greater than the pivot are on the right.

As an example, here's how we rearrange the array:

• At the pivot element, a pointer is fixed. Beginning with the first index, the pivot element is compared to the elements.

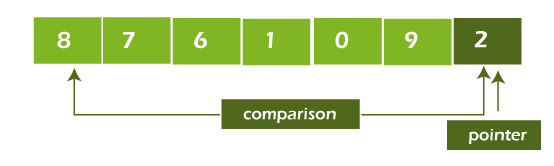
• A second pointer is set for the element if it is larger than the pivot element.

• The pivot is now being compared to other elements. If a smaller element than the pivot element is found, the smaller element is swapped with the larger element discovered earlier.

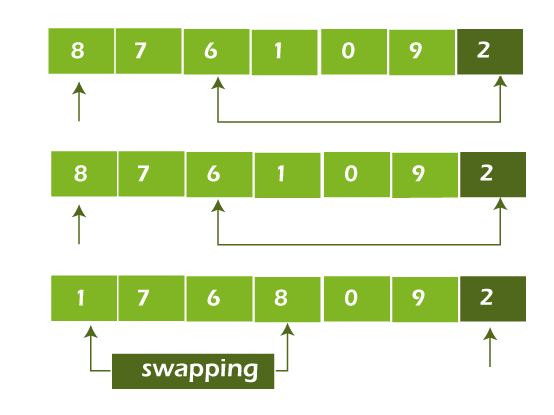
• The process is repeated to set the second pointer to the next greater element. Also, replace it with a smaller element.

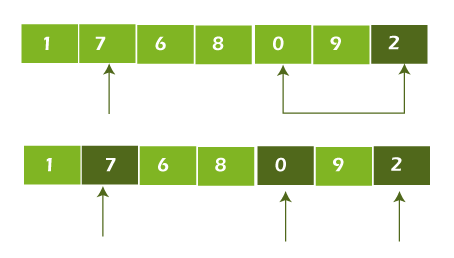
• The procedure continues until the second-to-last element has been reached.

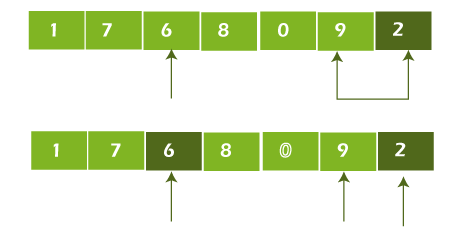
• The pivot element is then replaced with the second pointer.

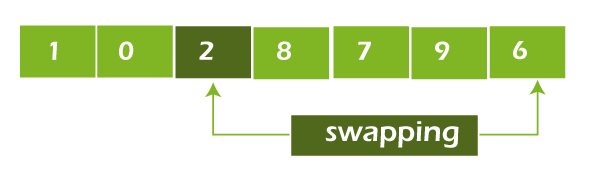
3. Separate Subarrays

Pivot elements are chosen separately for the left and right sub-parts. Step 2 is then repeated.

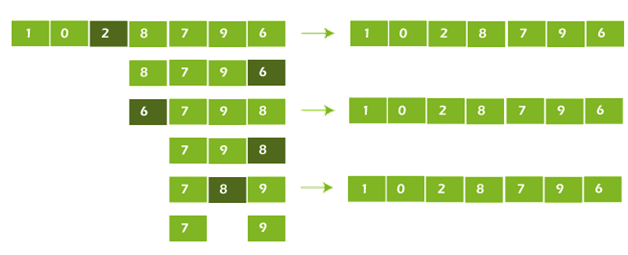
The subarrays are subdivided until each subarray consists of only one element. The array is already sorted at this point.

Algorithm of Quick Sorting

In order to sort an array of n elements in increasing order, use the following commands:

quickSort(array, leftmostIndex, rightmostIndex)
  if (leftmostIndex < rightmostIndex)
    pivotIndex <- partition(array,leftmostIndex, rightmostIndex)
    quickSort(array, leftmostIndex, pivotIndex - 1)
    quickSort(array, pivotIndex, rightmostIndex)


partition(array, leftmostIndex, rightmostIndex)
  set rightmostIndex as pivotIndex
  storeIndex <- leftmostIndex - 1
  for i <- leftmostIndex + 1 to rightmostIndex
  if element[i] < pivotElement
    swap element[i] and element[storeIndex]
    storeIndex++
  swap pivotElement and element[storeIndex+1]
return storeIndex + 1

The Quicksort Algorithm is depicted visually.

The illustrations below will help you understand how the quicksort algorithm works.

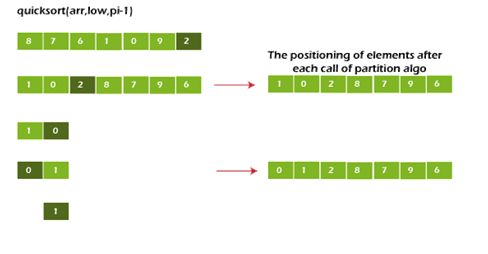
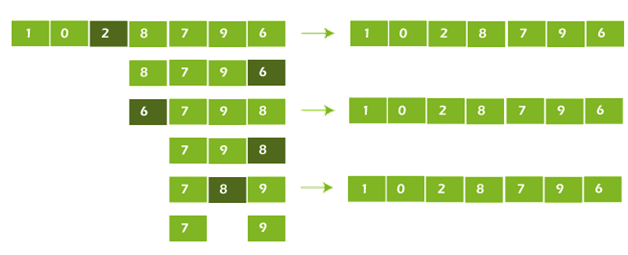
Code for Quick Sort in C

// Quick sort in C


#include <stdio.h>


// function to swap elements
void swap(int *a, int *b) {
  int t = *a;
  *a = *b;
  *b = t;
}


// function to find the partition position
int partition(int arr[], int low, int high) {
  
  // select the rightmost element as pivot
  int pivot = arr[high];
  
  // pointer for greater element
  int i = (low - 1);


  // traverse each element of the arr
  // compare them with the pivot
  for (int j = low; j < high; j++) {
    if (arr[j] <= pivot) {
        
      // if element smaller than pivot is found
      // swap it with the greater element pointed by i
      i++;
      
      // swap element at i with element at j
      swap(&arr[i], &arr[j]);
    }
  }


  // swap the pivot element with the greater element at i
  swap(&arr[i + 1], &arr[high]);
  
  // return the partition point
  return (i + 1);
}


void quickSort(int arr[], int low, int high) {
  if (low < high) {
    
    // find the pivot element such that
    // elements smaller than pivot are on left of pivot
    // elements greater than pivot are on right of pivot
    int pi = partition(arr, low, high);
    
    // recursive call on the left of pivot
    quickSort(arr, low, pi - 1);
    
    // recursive call on the right of pivot
    quickSort(arr, pi + 1, high);
  }
}


// function to print arr elements
void printArr(int arr[], int size) {
  for (int i = 0; i < size; ++i) {
    printf("%d  ", arr[i]);
  }
  printf("\n");
}


// main function
int main() {
  int arr[] = {8, 7, 2, 1, 0, 9, 6};
  
  int n = sizeof(arr) / sizeof(arr[0]);
  
  printf("Unsorted Array\n");
  printArr(arr, n);
  
  // perform quicksort on arr
  quickSort(arr, 0, n - 1);
  
  printf("Sorted array in ascending order: \n");
  printArr(arr, n);
}

The following output should be generated by this programme:

Output

Array Sorted in Ascending Order:
-12 -9 0 17 46

Complexity for Quick Sort

Time Complexity
Best Case	O(n log n)
Worst Case	O(n2)
Average	O(n log n)
Space Complexity	O(log n)
Stability	No

Complexities of Time

• Complexity in the worst-case scenario: O (n²)

When the pivot element chosen is either the greatest or the smallest, this occurs.

As a result of this condition, the pivot element ends up at the very end of the sorted array. One sub-array is always empty, while the other has n - 1 elements. As a result, quicksort is only applied to this sub-array.

For scattered pivots, however, the quicksort algorithm performs better.

• Complexity in the Best-Case Scenario: O (n log n)

It happens when the pivot element is always in the middle or close to the middle.

• Case Complexity on the Average: O (n log n)

It occurs when none of the above conditions are met.

Complexity of Space

Quick sort has a space complexity O (log n).

Applications for Quick Sorting

When the Quicksort algorithm is used,

• Recursion is supported by the programming language.
• It is important to consider the complexity of time.
• It is important to consider the complexity of space.